
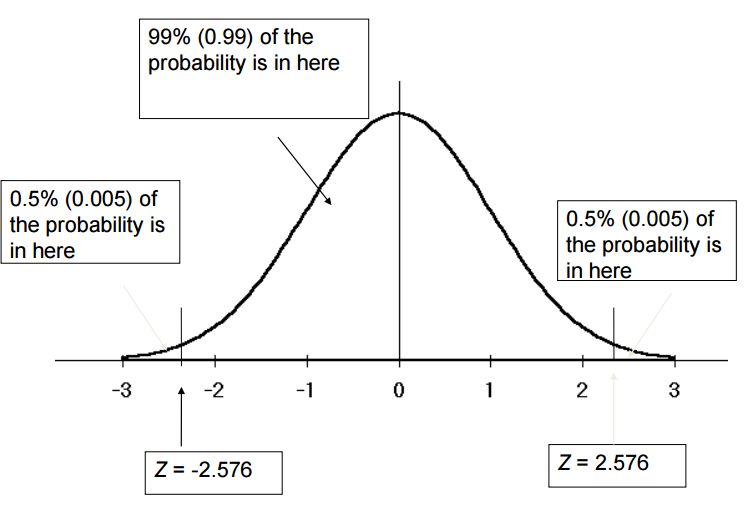
The three worst errors in statistics are (in order, starting with the worst). (123, 456) is a 95% confidence interval for μ Third there is the parameter being estimated, That the confidence interval actually contains the parameter.Ī confidence interval has three elements. Probability (conventionally expressed as a percent, though this is archaic) The confidence level of a confidence interval is the If measurements were available for the whole population). (a number that could, in theory, be calculated from the population,
98 CONFIDENCE INTERVAL Z SCORE HOW TO
For some further information, see our blog post on The Importance and Effect of Sample Size and for guidance on how to choose your sample size for estimates of the population mean, see our sample size calculator. Choosing a sample size is an important aspect when desiging your study or survey. The larger the sample size, the more certain you can be that the estimate reflects the population. This is the total number of samples randomly drawn from you population. The higher the confidence level the more certain you can be that the interval contains the true mean.

If the study was repeated and the range calculated each time, you would expect the true value to lie within these ranges on 95% of occasions. The confidence level is the probability that the confidence interval contains the true population mean. Μ is the sample mean, n is the sample size and x 1,…,x n are the n sample observations. The sample standard deviation is calcuated as s=√ σ 2, where: Where n is the sample size and x 1,…,x n are the n sample observations. The sample mean is your ‘best guess’ for what the true population mean is given your sample of data and is calcuated as: However, the relationship is not linear, e.g., doubling the sample size does not halve the confidence interval. The larger your sample size, the more certain you can be that the estimate reflects the population, so the narrower the confidence interval. DiscussionĬalculating a confidence interval provides you with an indication of how reliable your sample mean is (the wider the interval, the greater the uncertainty associated with your estimate).īy changing the four inputs (the sample mean, sample standard deviance, confidence level and sample size) in the Alternative Scenarios, you can see how each input is related to the confidence interval. for a confidence level of 95%, α is 0.05 and the critical value is 1.96), μ is the sample mean, s is the sample standard deviation, n is the sample size and N is the population size. Note that a Finite Population Correction (FPC) has been applied to the confidence interval formula. Z α/2 is the critical value of the Normal distribution at α/2 (e.g. This calculator uses the following formula for the confidence interval, ci: If the blood pressure of a further 900 adults were measured then this confidence interval would reduce to between 69.51 and 70.49mmHg (assuming the estimated mean and standard deviation remained the same).
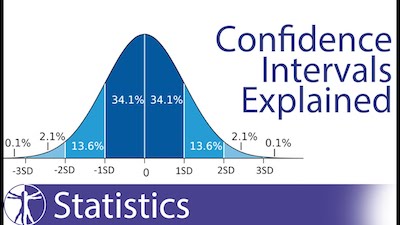
Using this information the 95% confidence interval is calculated as between 68.43 and 71.57mmHg. From their sample, they estimate the sample mean to be 70mmHg and the sample standard deviation to be 8mmHg. A study aims to estimate the mean systolic blood pressure of British adults by randomly sampling and measuring the blood pressure of 100 adults from the population.


 0 kommentar(er)
0 kommentar(er)
